This article is part of a set of three; the common factor is Calculus of Variations. In classical physics Calculus of Variations is applied in three areas: Optics, Statics, and Dynamics. Discussion of application in statics is included in the 'Foundation' article.
The other two articles:
| Foundation: | Calculus of Variations | |
| Optics: | Fermat's stationary time |
I strongly recommend to first absorb the contents of the 'Calculus of Variations' article.
The Energy-Position equation
1. From F = ma to the Work-Energy theorem
2. From Work-Energy to stationary action
3. Trial trajectories: response to variation
3.1 Potential increases linear with height
3.2 Potential increases quadratic with displacement
3.3 Potential increases proportional to cube of displacement
Appendix I The meaning of stationary
Appendix II Jacob's Lemma
This exposition is about Hamilton's stationary action. The usual presentation is to posit Hamilton's stationary action, and to proceed with showing that F = ma can be recovered from it.
However, it is also possible to proceed in the other direction. You can start with F = ma, and proceed to Hamilton's stationary action in all forward steps. The development is in two stages:
- Derivation of the Work-Energy theorem from F = ma
- Demonstration that in cases where the Work-Energy theorem holds good Hamilton's stationary action will hold good also.
The Work-Energy theorem and Hamilton's stationary action have the following in common: the physics taking place is described in terms of kinetic energy and potential energy. As an overarching name for both the Work-Energy theorem and Hamilton's stationary action I will use the expression 'energy mechanics'.
Interactive diagrams
The intention of this article is to let the interactive diagrams tell the story. You can choose to jump ahead to the interactive diagrams However, I strongly recommend you return to these paragraphs at some point; for overall understanding they are necessary.
Requirement for well defined potential energy
Given a particular force: in order to have a well defined expression for potential energy the force must have the property that the work done in moving from some point A to some point B is independent of the path taken between the two points. The validity of energy mechanics is limited to forces with that property.
(It may be that in terms of a deeper theory such as quantum physics all interactions actually have that potential-is-independent-of-the-path property, but we should not blindly assume that.)
As we know: the principle of conservation of energy is asserted without any restriction. That's why the concept is referred as a 'principle', it is a blanket statement.
The discussion in this article avoids the blanket statement. The discussion in this article is limited to the classes of cases where by way of experimental corroboration it is evident that the work done in moving from some point A to some point B is independent of the path taken between the two points.
1. From F = ma to the Work-Energy theorem
In preparation for deriving the work-energy theorem we first derive a kinematical relation between the integral of acceleration with respect to position, and velocity.
In the course of the derivation the following two relations will be used:
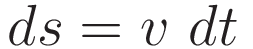
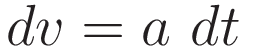
Let a represent an arbitrary acceleration profile. We set up integration of this acceleration profile from a starting point s0 to a final point s
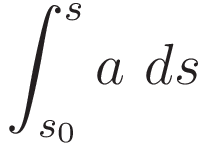
Intermediary step: change of the differential according to (1.1), with corresponding change of limits.
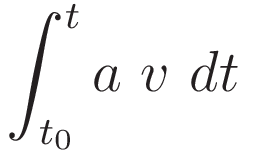
Change the order:
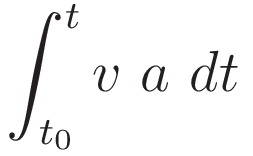
Change of the differential according to (1.2), with corresponding change of limits.
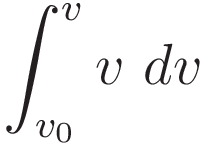
So we have:
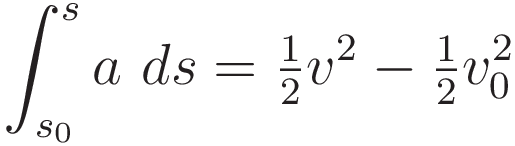
I want to emphasize just how general the validity of (1.7) is: it follows from the definitions (1.1) and (1.2) and the mathematics of differentiation/integration. That is: (1.7) obtains mathematically, independent from any physics.
The Work-Energy theorem
We start with the force-acceleration principle:
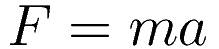
Specify integration with respect to the position coordinate:
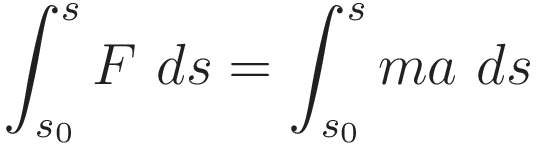
Use (1.7) to process the right hand side. The result is the work-energy theorem:
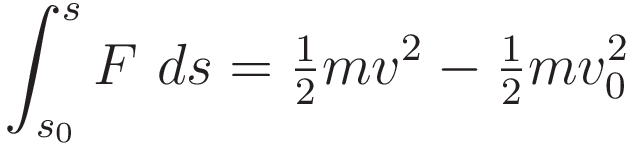
The work-energy theorem motivates the definitions of potential energy and kinetic energy.
Historically: for several centuries, from the time of Huygens to the time of Hamilton, the concept of 'living force' was used, which was defined as mv². It was in the 1850's that the physics community shifted to a new name and a new definition: ½mv². The benefit of that shift: by defining kinetic energy as an integral (integral of ma with respect to the position coordinate) the concept of kinetic energy is defined in terms of the work-energy theorem.
To emphasize the structure of the Work-Energy theorem I give the elements arranged in a table. In each row the statement follows mathematically from the statements in the row(s) above it.
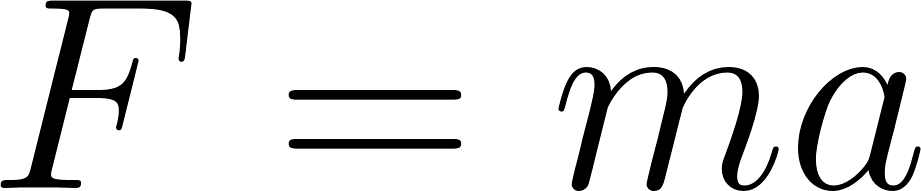
|
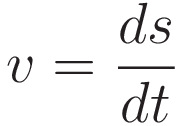
|
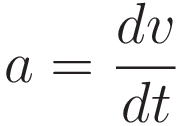
|
|
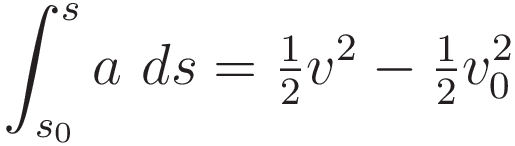
|
|||
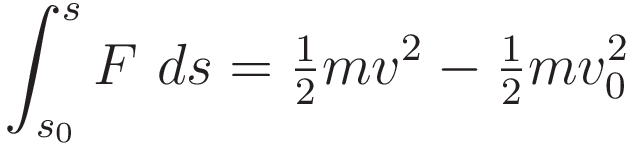
|
|||
Potential energy
Historically the concept of potential energy arose before the work-energy theorem reached today's standard form. With the benefit of hindsight we recognize that the concept of potential energy capitalizes on the work-energy theorem.
Potential energy is defined as the negative of the left hand side of (1.9); the integral of force over distance.
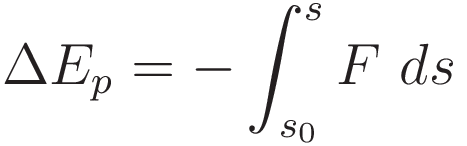
In order for the potential energy to be well defined a specific condition must be met: the value of the integral from point s0 to point s must be independent of how the test object moves from point s0 to point s.
When the potential energy is well defined:
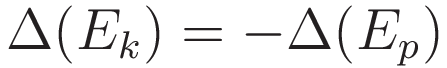
That is: with potential energy defined according to (1.10): when an object is moving down a potential gradient the kinetic energy will increase by the amount that the potential energy decreases. (With everything reversed when moving in the opposite direction, of course.)
(1.12) combines three statements. I present these three statements as a unit to emphasize the interconnection between them. While the statements are different mathematically, the physics content of these three is the same.
![\begin{array}{rcl}
F & = & ma \\[+10pt]
\int_{s_0}^s F \ ds & = & \tfrac{1}{2}mv^2 - \tfrac{1}{2}mv_0^2 \\[+10pt]
-\Delta E_p & = & \Delta E_k
\end{array}](../action_img/20211019_185500_690x301.png)
2. From Work-Energy to stationary action
Visual demonstration
graphlet 2.1 offers a visual representation of the connection between the work-energy theorem and Hamilton's stationary action
In the section following this section the connection will be given in equation form.
Black curve: trajectory
Red: kinetic energy
Green: work done
The upturned parabola in the upper left sub-panel represents height as a function of time, of an object that is thrown vertically upward. There is a vertically downward acting force. On the ascent the object is decelerated, followed by acceleration back down.
The slider at the bottom of the diagram represents sweeping out variation. When the variation is at the value zero the trial trajectory coincides with the true trajectory.
Note especially that variation of a trial trajectory is exclusively variation of position coordinate. As a consequence: differentiation with respect to variation can always be re-expressed as differentiation with respect to position coordinate.
The following three relations are plotted:
upper-left subpanel: height of the trial trajectory as a function of time
upper-right and lower-left: Kinetic energy (red) and Work done (green) as a function of time
lower-right: the value of the integral-with-respect-to-time as a function of variational parameter pv.
Note especially that the green curve represents work done. Since potential energy is defined as the negative of work done the green curve represents minus potential energy. Stated differently: the green curve represents potential energy, but with the value mirrored in the horizontal axis. Mirroring the curve for the potential energy helps for the visualization.
Use the slider to sweep out variation.
As you move the slider:
· Upper-left: The height of the trial trajectory changes linear with the slider position.
· Upper-right and lower-left: the shape of the curve for each energy changes; for the respective energies the rate of change is different.
· Lower-right:
·Green curve: the value changes linear with the variation.
·Red curve: the value changes quadratically.
Now focus on the lower-right subpanel, which displays the value of the integral as a function of the variational parameter pv. The moving dot represents the integral value that corresponds to the current pv value. Examine the point in variation space where the trial trajectory coincides with the true trajectory: at that point the rate of change of the red integral value matches the rate of change of the green integral value.
If the potential energy would have been plotted directly (rather than mirroring it) then as you sweep out variation you would see the potential energy and kinetic energy co-changing. In the actual diagram the green dot and the red dot are counterchanging (in response to variation), because of the mirroring.
The lower-right subpanel has a checkbox labeled 'sum'. With that checkbox checked the sum of the two integrals is displayed, in the color blue. At the point in variation space where the trial trajectory coincides with the true trajectory: at that point the derivative of the blue curve is zero.
The usual notation for Hamilton's action is to represent it with the letter S. In the graphlet the red integral has the label Sk for 'kinetic energy component of the action S'. Potential energy is defined as the negative of work done, hence the green integral has the label '-Sk' for 'potential energy component of the action S'. The blue curve represents Hamilton's action:
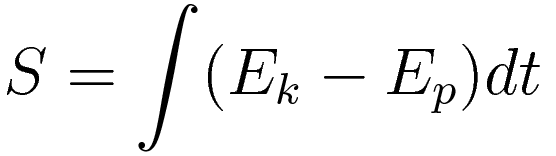
Derivative with respect to position.
This section picks things up from equation (1.12), building onto it by making use of the fundamental theorem of Calculus: differentiation and integration are each other's inverse.
In section 1. the derivation of the work-energy theorem from F=ma was by way of integration with respect to the position coordinate. The following equation specifies the inverse: differentiation with respect to the position coordinate
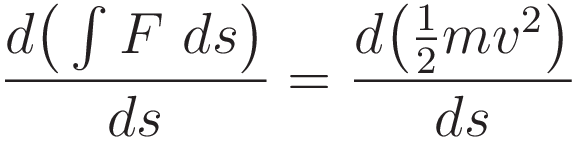
Given that differentiation is the inverse of integration we know in advance that processing this differentiation will recover F=ma.
A compact descriptive name of for (2.2) is 'energy-position equation'; this equation takes the derivative of energy with respect to position
About the right hand side of (2.3), the differentiation of kinetic energy with respect to position coordinate. That differentiation can be restated in a way that makes it easier to process. The following two differentiation operations are equivalent:
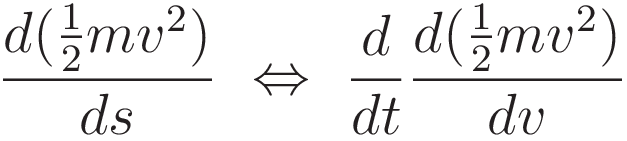
At first sight it may seem implausible that the two sides of (2.3) are equivalent.
Corroboration:
(2.3b) evaluates the right hand side of (2.3), and (2.3c) evaluates the left hand side of (2.3); both evaluate to ma, the product of mass and acceleration:
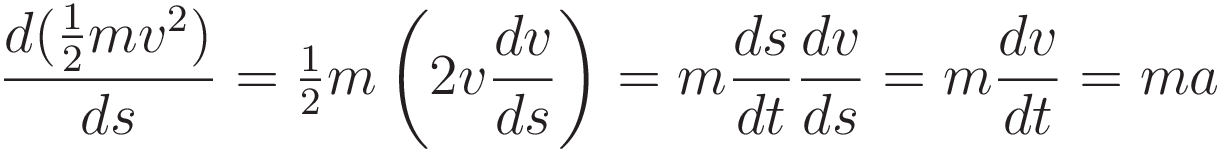
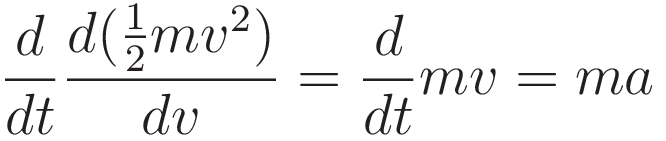
(2.4) is a restatement of (2.2), with the right hand side restated according to (2.3)
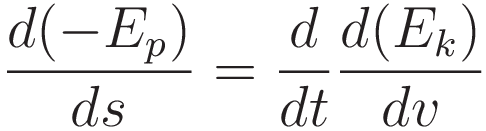
Rearrange to have both terms on the same side:
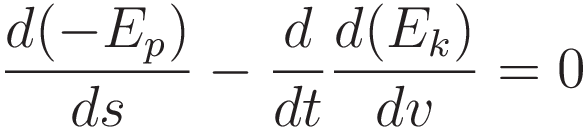
The following notation says the same thing as (2.5) does, since ∂Ek/∂s=0, and ∂Ep/∂v=0.
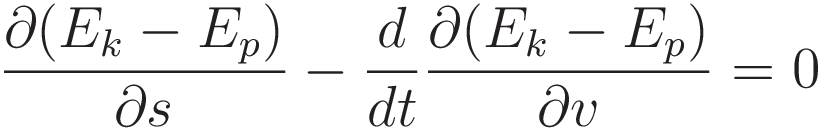
(2.6) is the equation that we get when the Lagrangian (Ek-Ep) is inserted in the Euler-Lagrange operator
In this section the property that is demonstrated visually in graphlet 2.1 is demonstrated in equation form.
The relation between Hamilton's stationary action and the work-energy theorem comes about through the property that differentiation and integration are linear operations.
As we know: the property of linearity means differentiation and integration are additive, we can move additions in and out.
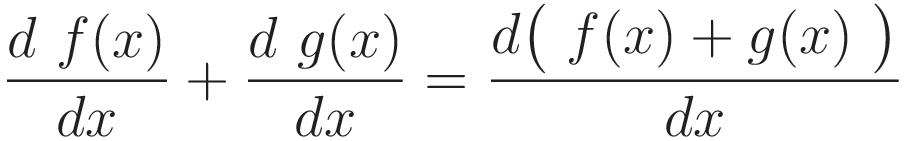
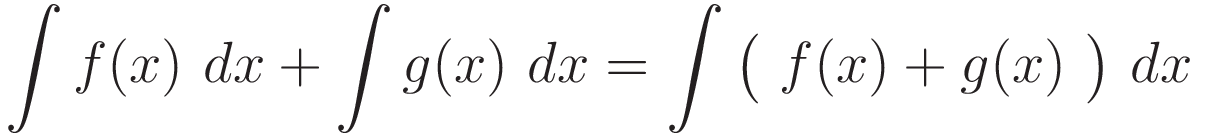
A specific instance of the above is that a multiplication factor can be moved in and out:
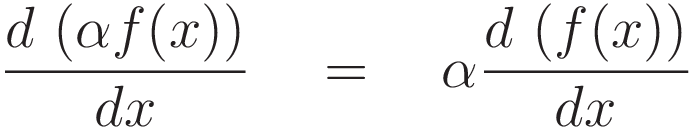
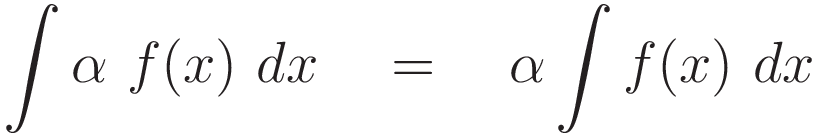
(2.11) is a repeat of (2.2). Reminder: (2.11) should not be thought of as a statement about a specific point along the trajectory. The condition is that (2.11) must be satisfied concurrently for the trajectory as a whole.
Upon sweeping out variation: at the point in variation space where (2.11) is valid (2.12) is valid also:
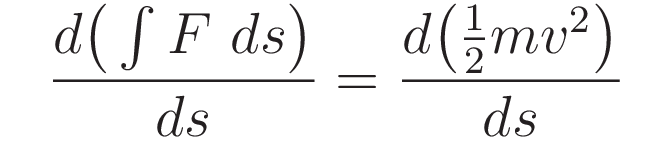
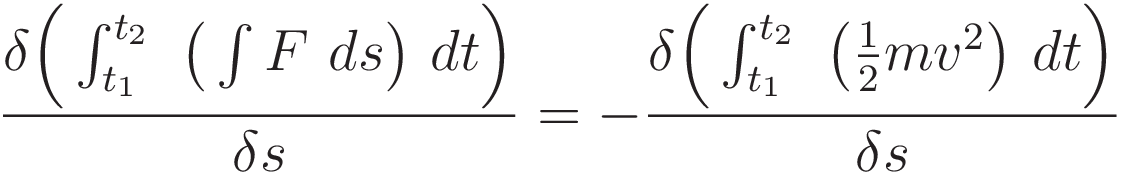
In (2.12) the  symbol is used to specify that the differentiation is differentiation with respect to variation.
symbol is used to specify that the differentiation is differentiation with respect to variation.
So: from (2.11) to (2.12):
- In (2.11) differentiation of a function with respect to position coordinate is specified; in (2.12) a time integration of the function has been has been interjected.
- On the right hand side a minus sign has appeared; I will get back to that.
Given a trajectory we can describe the kinetic energy as a function of time, and the potential energy as a function of time. The true trajectory has the property that the derivative of the kinetic energy function matches the derivative of the potential energy function, as expressed by (2.11). That is: the true trajectory has the property that the slope of the kinetic energy function matches the slope of the potential energy function.
That property of matching rate of change carries over from (2.11) to (2.12); the property propagates to the integral. When the slope of a function f increases with a factor α the integral of that function increases with that factor α
About application of variation:
In the case of Hamilton's stationary action: the direction of the variation is intrinsically in the direction of the position coordinate. That is why differentiation with respect to variation can be re-expressed as differentiation with respect to position coordinate.
The minus sign
As mentioned earlier: in (2.12) the right hand side has a minus sign. The minus sign is there because the transition from (2.11) to (2.12) is a transition from co-changing to counter-changing.
(2.11) expresses following motion over time (even though differentiation with respect to the position coordinate is specified). When followed over time: work done and kinetic energy are co-changing.
(2.12) is stated in terms of differentiating with respect to variation. When variation is applied work done and kinetic energy are counter-changing.
Because of the transition from co-changing to counter-changing a minus sign must be added. The choice on which side of the equals sign to add the minus sign is arbitrary. In this case I chose to add the minus sign on the right hand side: it was typographically convenient.
In (2.13):
(∫ F ds) has been replaced with -Ep
(½mv²) has been replaced with Ek
the term with the kinetic energy has been moved to the other side of the equals sign.
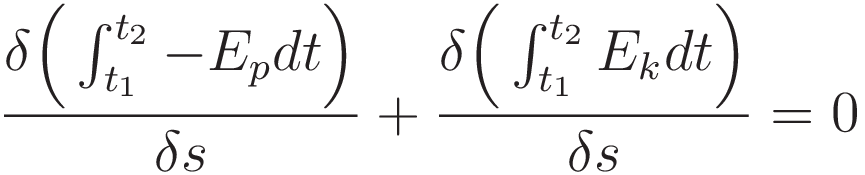
In (2.14) the two terms of (2.13) have been combined into a single term; (2.14) expresses Hamilton's stationary action.
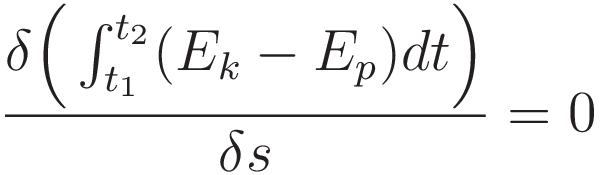
Discussion
Hamilton's stationary action expresses the following: the true trajectory has the property that everywhere along the trajectory the rate of change of kinetic energy matches the rate of change of potential energy.
The way to understand Hamilton's stationary action is to think of it in terms of (2.13): a criterion that two rates of change should match. The way to understand (2.13) is to think of it in terms of (2.11).
3. Trial trajectories: response to variation
The following three graphlets are three instances of the same graphlet, for three successive classes of cases.
As you manipulate the sliders: the process to home in on the true trajectory is to manipulate the trial worldline such that over the entire trajectory the slope of the kinetic energy curve (red) matches the slope of the potential energy curve (green). When the trial trajectory is at thet the point in variation space such that those two slopes match the derivative of Hamilton's action is zero.
3.1 Potential increases linear with height
Potential energy increases linear with height; the trajectory is a parabola
Graphlet 3.1 is for the case of a uniform force, causing an acceleration of 2 m/s2.
As we know: with a uniform force the curve that represents the height as a function of time is a parabola.
In the 'energy' sub-panel the green curve represents the minus potential energy. In effect the potential energy curve has been flipped upside down. That way you can see directly that when the trial trajectory coincides with the true trajectory the red and green curve are parallel to each other everywhere.
(Any form of calculating the true trajectory uses the derivative of the potential energy. That is, in calculation the value of the potential energy itself is not used. Because of that the choice of zero point of potential energy is arbitrary.)
In the lower right subpanel:
The blue dot represents Hamilton's action.
The label of the horizontal axis is pv, which stands for 'variational parameter'. The positioning of the dots corresponds to the value of the main slider.
In the case of linear potential: when the trial trajectory coincides with the true trajectory the value of Hamilton's action is minimal/minimized. In the case implemented in this graphlet: any change of the trial trajectory results in raising the value of Hamilton's action.
3.2 Potential increases quadratic with displacement
Potential energy increases quadratic with displacement
Graphlet 3.2 is for the case where the force increases in linear proportion to the displacement. This case is commonly referred to as Hooke's law.
As we know: With Hooke's law the resulting motion is harmonic oscillation and the curve that represents the displacement as a function of time is the sine function.
With Hooke's law the potential energy increases with the square of the displacement. That is: with Hooke's law we have that the rate of change of potential energy is on par with that of the kinetic energy: with Hooke's law the expressions for the energies are both quadratic expressions.
As we know: with Hooke's law the amplitude and period of the resulting oscillation are independent from each other. Hamilton's stationary action corroborates that: when the trial trajectory is the harmonic oscillation function then if you change the amplitude the value of the action remains the same.
(In the diagram the actual curve is the cosine function; the point is that it is the harmonic oscillation function.)
3.3 Potential increases proportional to cube of displacement
Potential energy proportional to cube of displacement.
Graphlet 3.3 is for the case where the force increases proportional to the square of the displacement, hence the potential energy increases with the cube.
Click the button 'Show numerical' to show how the curve displayed in graphlet 6 was independently verified
Comparing the trajectories
In graphlet 3.1 the potential energy as a function of position is linear. Whenever the potential energy as a function of position is of lower order than the expression for the kinetic energy the action reaches a minimum when the derivative of the action is zero. In graphlet 3.2 the potential energy function and the kinetic energy function are both quadratic expressions, so the action is neither a minimum nor a maximum. Here in graphlet 3.3 the potential energy as a function of position is a higher order expression than the one for the kinetic energy (cubic versus quadratic), and consequently when the derivative of the action is zero the action reaches a maximum.
I recommend enlarging the graphlet to the full width of the browser window. Visibility of the navigation column of this page can be toggled. When the navigation column has been hidden: use the button 'Larger' and zoom in on the page as a whole to make the graphlet fill the entire width of the browser window.
How to operate the demonstration:
The graphlet set 3.1, 3.2, and 3.3 is designed to be discoverable, but for the sake of completeness I provide a description.
The main slider, located at the bottom of the graphlet, executes a global variation sweep. The value displayed in the "knob" of the main slider is a value to implement the variation, from here on I will refer to it as the 'variational parameter pv' In the 'integral' panel the variational parameter pv is along the horizontal axis.
Names for the 4 sub-panels:
- upper left: Control panel
- lower left: Height panel
- upper right: Energies panel
- lower right: Integrals panel
Note: The 3 sub-panels with a coordinate system are named after their vertical axis name: Height, Energy, Integral.
In the starting configuration the trajectory points (height panel) have been placed such that they coincide (to a very good approximation) with the true trajectory of the object.
When the object is moving along the true trajectory the rate of change of kinetic energy matches the rate of change of potential energy (work-energy theorem). This match of rate of change has been emphasized by turning the graph of the potential energy (green) upside down. When the object is moving along the true trajectory the red and green curve have the same slope along the entire trajectory.
The energies panel is where it happens. The energies panel represents how the equation that you are using solves for the true trajectory.
Hamilton's Action
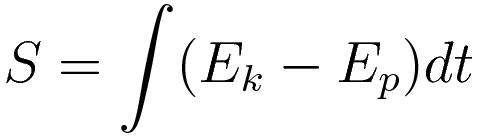
Hamilton's Action is represented by the blue dot in the Integrals panel. Use the main slider to sweep out variation. The blue dot follows a curve; it is a curve in variation space. The slope of that curve represents the derivative of Hamilton's Action with respect to variation.
At the point where over the entire trajectory the derivative of the kinetic energy is equal to the derivative of the minus potential energy the derivative of the blue dot is zero.
In the control panel the two sliders on the far left are adjusters for the trajectory. The upper adjuster morphs the trajectory towards a curve that is more blunt than the true trajectory; the lower adjuster morphs the trajectory towards a triangle shape.
These adjusters allow morphing of the trial trajectory while maintaining that during the entire ascent the velocity never reverses from decreasing to increasing again, which would be unphysical.
The row of ten sliders is for adjustment of individual nodes. The three radio buttons toggle between three sets of node adjusting sliders.
The '× 1' button: for a ratio of 1-to-1 of moving the slider and movement of the corresponding node of the trajectory.
The '× 0.1' button: ratio of 10 to 1
The '× 0.01' button: ratio of 100 to 1, for fine adjustment.
The button 'Consolidate':
Clicking the button 'Consolidate' does the following: the current value of the '× 0.1' slider is transferred to the '× 1' slider. That is: after clicking the button 'Consolidate' the position of the '× 0.1' slider is reset to its zero position, and the '× 1' slider has been incremented accordingly.
In the energies panel: the grey point is draggable, and it drags the entire curve of the kinetic energy (red) with it. By shifting the kinetic energy curve over to the potential energy curve the user can verify that the red and green curve are parallel to each other along the entire trajectory. (The evaluation of the integral uses the unshifted position of the kinetic energy curve.)
In the integrals panel:
· Red/Green point: value of the integral of the red/green curve of the energies panel
· Blue point: the sum of the values of the red point and the green point
Finding the true trajectory by adjusting the sliders
As a seed move the '× 1' sliders so that the nodes are positioned along a straight line, say at 45 degrees.
In the 'Energies' subpanel, move the graph of the kinetic energy (red dots) down, on average a bit below the potential energy graph (green dots)
Use the '× 0.1' sliders to move the nodes upward bringing the red dots and green dots into alignment.
The true trajectory has the property that the rate of change of kinetic energy matches the rate of change of potential energy, so you want to make those two curves parallel to each other.
Appendix I: The meaning of 'stationary'
At the x-coordinate where the red and green curve have equal slope the blue curve is at zero slope.
Red and green are both ascending functions. We want to identify the coordinate where the rate of change of the red value matches the rate of change of the green value. The shortest way to get there is by setting up an equation with the derivative of the red curve on one side and the derivative of the green curve on the other side.
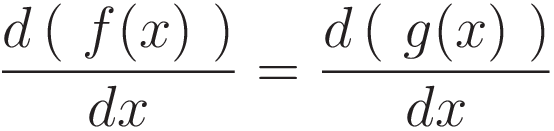
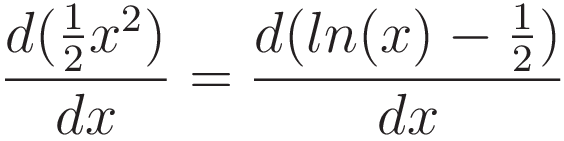
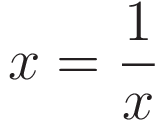
In the case of Hamilton's stationary action the same comparison is performed, but in a form that takes more steps. We see the following: a third function is defined, which is called the Lagrangian L: L=(Ek-Ep), and the point to be identified is called the point of 'stationary action'. 'Stationary action' is another way of saying: identify the point where the derivative of the Lagrangian is zero.
Summerizing:
Hamilton's stationary action works by imposing the constraint that was established with (1.11): the rate of change of kinetic energy must match the rate of change of potential energy.
Appendix II: Jacob's Lemma
When Johann Bernoulli had presented the Brachistochrone problem to the mathematicians of the time Jacob Bernoulli was among the few who was able to find the solution independently. The treatment by Jacob Bernoulli is in the Acta Eruditorum, May 1697, pp. 211-217
Jacob opens his treatment with an observation concerning the fact that the curve that is sought is a minimum curve.
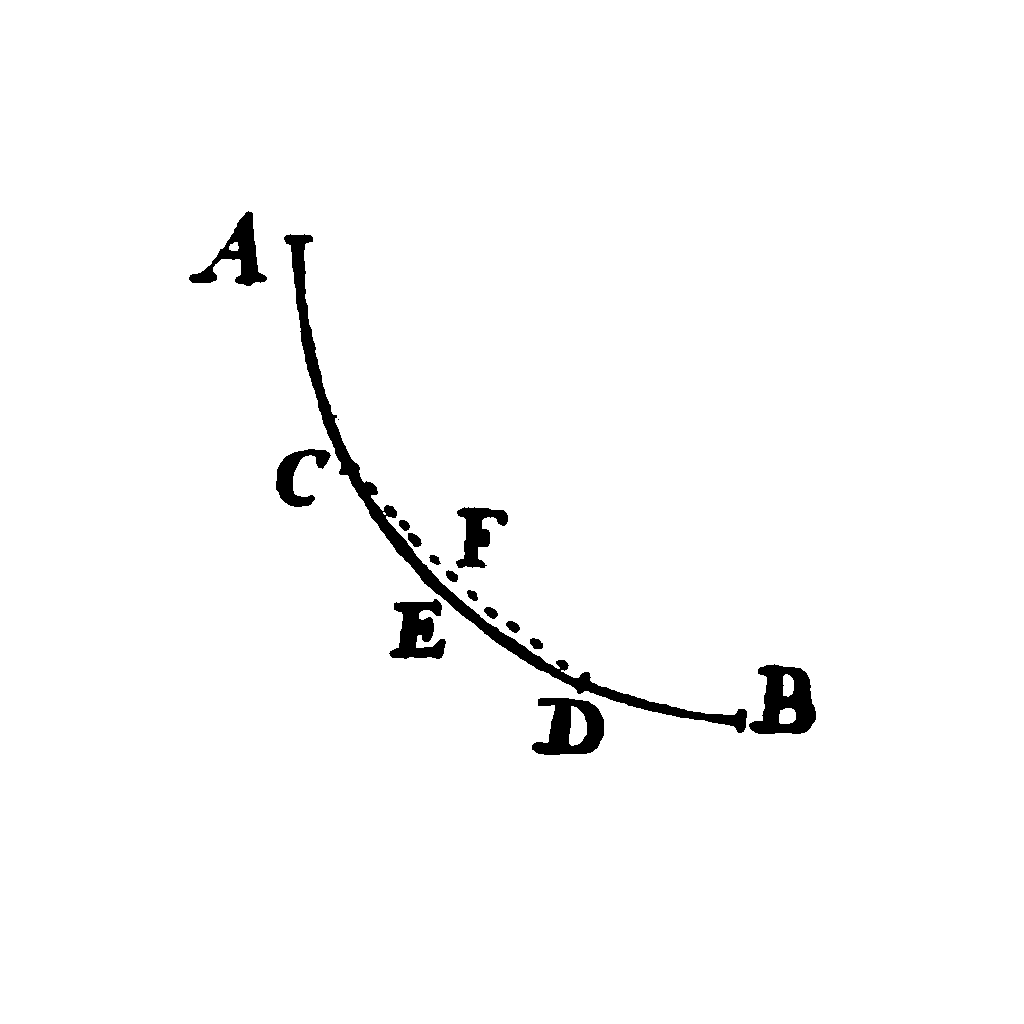
Lemma. Let ACEDB be the desired curve along which a heavy point falls from A to B in the shortest time, and let C and D be two points on it as close together as we like. Then the segment of arc CED is among all segments of arc with C and D as end points the segment that a heavy point falling from A traverses in the shortest time. Indeed, if another segment of arc CFD were traversed in a shorter time, then the point would move along ACFDB in a shorter time than along ACEDB, which is contrary to our supposition.
Jacob's lemma generalizes to all cases where the curve that you want to find is an extremum; either a maximum or a minimum. If the evaluation is an extremum for the entire curve, then it is also an extremum for any sub-section of the curve, down to infinitesimally short subsections.
The interactive diagrams on this page are created with the Javascript Library JSXGraph. JSXGraph is developed at the Lehrstuhl für Mathematik und ihre Didaktik, University of Bayreuth, Germany.

This work is licensed under a Creative Commons Attribution-ShareAlike 3.0 Unported License.
Last time this page was modified: May 31 2025